Interaction between Two Straight Lines

The vertices of a triangle $∆OBC$ are $O \left(0, 0\right), B\left(-3, -1\right), C\left(-1, -3\right)$ find the equation of the line parallel to $BC$ and intersecting the sides $OB \& OC$, whose perpendicular distance from the point $\left(0, 0\right)$ is half.

A straight line L is perpendicular to the line 5x - y = 1. The area of the triangle formed by the line L & the co-ordinate axes is 5. Find the equation of the line.

Equation of the line passing through $(1,2)$ and parallel to the line $y=3x-1$ is

Two vertices of a triangle are $\left(3,-2\right)$ and $\left(-2,3\right)$, and its orthocentre is $\left(-6,1\right)$. Then, the third vertex of this triangle cannot lie on the line

The distance between the lines $4x+3y-11=0$ and $8x+6y-15=0$ is $\frac{a}{10}$ units. Write the value of $a$.

The $y$-intercept of the line passing through $A(6,1)$  and perpendicular to the line $x-2y=4$ is …

Distance between two parallel lines $y=2x+4$ and $y=2x-1$ is

If the lines $kx-2y-1=0$ and $6x-4y-m=0$ are identical (co -incident) lines, then the values of $k$ and $m$ are ......

The distance between lines $x+y+2=0$ and $3x+3y-7=0$ is

The $x$-axis, $y$-axis and line passing through point $A(5,0)$ form a triangle $AOB$, where $O$ is origin. If $\angle A={60}^o$, then the area of triangle in square units is

Distance between the lines $5x+3y-7=0$ and $15x+9y+14=0$ is

The measure of the angle between pair of lines $x=y$ and $y=0$ is?

A  new airport, $S$ is to be constructed at some point along a straight road, $R$, such that its distance from a nearby town, $T$, is the shortest possible.

The town, $T$, and the road, $R$, are placed on a coordinate system where $T$ has coordinates $(80, 140)$ and $R$ has equation $y=x-80$. All coordinates are given in kilometres.

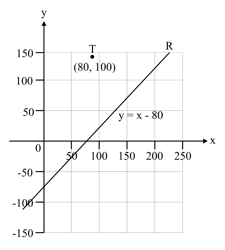
Determine the coordinates of $S$, the new airport.

Lines $L_1$ and $L_2$ are given by the equations  $L_1:ax-3y=9$ and $L_2:y=\frac{2}{3}x+4.$

The two lines are perpendicular.Hence, determine the coordinates of the intersection point of the lines.

The line $L$ has equation $y=3x-5$. For the line $x+3y+9=0$, State with reasons whether they are parallel to $L$, perpendicular to $L$, or neither.

The line $L$ has equation $y=3x-5$. For the line $y=\frac{-1}{3}x+4$, State with reasons whether they are parallel to $L$, perpendicular to $L$, or neither.

The line $L$has equation $y=3x-5$. For the line $y-5=2(x-7)$, State with reasons whether they are parallel to $L$, perpendicular to $L$, or neither.

The line $L$ has equation $y=3x-5$. For the line$-6x+2y+8=0$,  State with reasons whether they are parallel to $L$, perpendicular to $L$, or neither.